The probability function is the random variable $$X$$ that associates a probability $$p_i$$ to every possible value of $$X$$ $$(x_1,x_2,\ldots,x_n)$$. We also need: $$$\begin{matrix}0 \leq p_i\leq 1 \\ p_1+p_2+p_3+ \ldots +p_n= \sum_{i} p_i=1\end{matrix}$$$
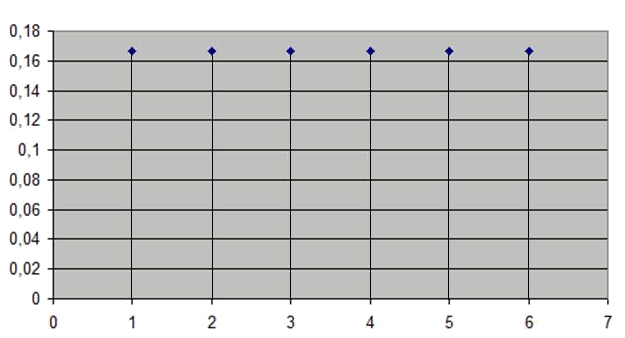
Let the random variable $$X$$ be the result of throwing a dice. Supposing that it has six equiprobable faces, the probabilities of every result are: $$$\displaystyle p(X=1)=P(X=2)=\ldots=P(X=6)=\frac{1}{6}$$$ It is possible to prove that we have $$$\displaystyle \sum_{i} p_i=6 \cdot \frac{1}{6}=1$$$ The graph of the above mentioned function is a bar chart: