Both the law of sines and the law of cosines are applicable to any kind of triangles, as opposed to the Pythagoras theorem that only applies to right-angled triangles.
Law of sines
The law of sines is a proportionality relation between the lengths of the sides of a triangle and the sines of the opposite angles. Given the triangle:
We have: $$$\displaystyle \frac{a}{\sin \alpha}=\frac{b}{\sin \beta}=\frac{c}{\sin \gamma}$$$
Knowing that two angles of a triangle are: $$A=30^\circ$$, $$B=45^\circ$$ and that the side opposite to the angle $$B$$ is $$b=\sqrt{2}$$ cm, we can calculate the side $$a$$ opposite to the angle $$A$$ by means of the law of sines. Let's see how:
First, we need to identify the information given in the problem. We have the following triangle:
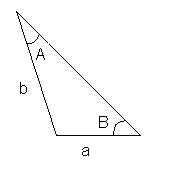
We know two angles and one side of this triangle. For one of the angles that we know, $$B$$, we also know the length of the opposite side, $$b$$. And the side we are looking for is the opposite to the other known angle, $$A$$. Therefore, in the equality of the law of sines $$$\displaystyle \frac{a}{\sin A}=\frac{b}{\sin B}$$$
we only have one unknown. The rest is information given in the problem. And so, we can compute: $$$\displaystyle a=\frac{b \cdot \sin A}{\sin B}=\frac{\sqrt{2} \cdot \sin 30}{\sin 45}=\frac{\sqrt{2}\frac{1}{2}}{\frac{\sqrt{2}}{2}}=\frac{2\sqrt{2}}{2\sqrt{2}}=1$$$
Law of cosines
The law of cosines can be understood like a generalization of Pythagoras theorem for any kind of triangle. In other words, if we apply the law of cosines to a right triangle we obtain the same result as in Pythagoras theorem. We will get a relationship between the length of one side and the length of the other two and with the cosine of the angle formed by them. Given the triangle,
We have: $$$a^2= b^2+c^2-2 \cdot b \cdot c \cdot \cos \alpha$$$ This can be applied to any of the sides, so we also have these two identities: $$$\begin{array}{rc} b^2&=&a^2+c^2-2\cdot a \cdot c \cdot \cos \beta \\ c^2 &=& a^2+b^2-2\cdot a \cdot b \cdot \cos \gamma \end{array}$$$
Let's suppose that we know three sides of a triangle, $$a=2, b=3, c=\sqrt{7}$$ and we want to know the angles. From the law of cosines we know: $$$c^2=a^2+b^2-2\cdot a \cdot b \cdot \cos \gamma \Rightarrow 7=4+9-2\cdot 2 \cdot 3\cdot \cos \gamma \Rightarrow $$$ $$$6= 12 \cdot \cos \gamma \Rightarrow \cos \gamma= \displaystyle \frac{1}{2} \Rightarrow \gamma =60 $$$
Applying the law of cosines again we can find the second angle: $$$b^2=a^2+c^2-2 \cdot a \cdot c \cdot \cos \beta \Rightarrow 9=4+7-2\cdot 2 \cdot \sqrt{7} \cdot \cos \beta \Rightarrow $$$ $$$a=4\sqrt{7}\cdot \cos \beta \Rightarrow \cos \beta=\displaystyle \frac{1}{2}\sqrt{7} \Rightarrow \beta=79.10^\circ$$$
Finally, using our knowledge that the sum of the angles of a triangle is $$180^\circ$$, we have $$$\alpha=180-\beta-\gamma=40.9^\circ$$$

